 |
 |
Mechanochemical models for dermal wound healing
A crucial aspect of wound healing concerns the mechanochemical interaction of cells with their external environment. Cells deform and remodel the extracellular matrix (ECM) on which they move, setting up mechanical signalling cues. In publication 62 we derive a mechanochemical model for dermal wound healing which consists of a coupled system of nonlinear differential equations describing the spatiotemporal evolution over time of fibroblast cell density, ECM density and displacement, and growth factor concentration. We show that the model exhibits travelling waves of cell densities moving into the wound space and that it captures key aspects of the wound healing process.
By analysing simplified versions of the model we show that the model can exhibit spatially-varying contracted states, and we apply it to certain disorders such as fibrocontractive and fibroproliferative diseases (publications 71, 89, 92) and suggest certain therapies to reduce keloid scar formation. We extend the model to include the effects of angiogenesis (publication 91).
 |
 |
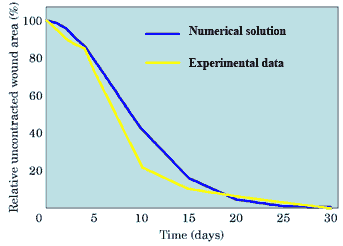
Comparison of model simulations and experimental data for wound closure (M.H. McGrath and R.H. Simon, Plast. Reconstr. Surg., 72, 66-72 (1983) and clearance of growth factor (K.H. Sprugel et al., Am. J. Pathol., 129, 601-613 (1987)). [Reproduced with permission from publication 62]
 |
Numerical solution of a wound that was initially on the interval [0,1] along the x-axis, with normal cell density (n=1) elsewhere. In this case cells not only fill in the wound space, but grow in uncontrolled fashion (denoted by black arrow and red lines) to a very high density and spatial extent much greater than the initial wound area. This is typical of a keloid scar. At a certain time in the simulation, we change a critical parameter determined by a bifurcation analysis and the system reverts to controlled healing (denoted by white arrow and green lines) reaching the normal healed steady state. [Reproduced with permission from publication 71]
** Work carried out in collaboration with L. Olsen and J.A. Sherratt.